Page 60 - Maths Class 05

LCM by Prime Factorisation Method

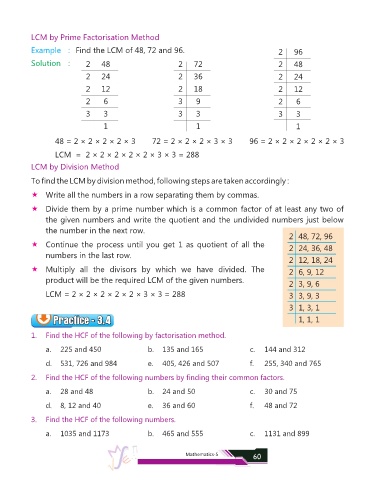
Example : Find the LCM of 48, 72 and 96. 2 96

Solution : 2 48 2 72 2 48

2 24 2 36 2 24
2 12 2 18 2 12

2 6 3 9 2 6

3 3 3 3 3 3
1 1 1

48 = 2 × 2 × 2 × 2 × 3 72 = 2 × 2 × 2 × 3 × 3 96 = 2 × 2 × 2 × 2 × 2 × 3

LCM = 2 × 2 × 2 × 2 × 2 × 3 × 3 = 288
LCM by Division Method

To find the LCM by division method, following steps are taken accordingly :

« Write all the numbers in a row separating them by commas.

« Divide them by a prime number which is a common factor of at least any two of

the given numbers and write the quotient and the undivided numbers just below
the number in the next row.
2 48, 72, 96
« Continue the process until you get 1 as quotient of all the
2 24, 36, 48
numbers in the last row.
2 12, 18, 24
« Multiply all the divisors by which we have divided. The 2 6, 9, 12

product will be the required LCM of the given numbers.
2 3, 9, 6
LCM = 2 × 2 × 2 × 2 × 2 × 3 × 3 = 288 3 3, 9, 3

3 1, 3, 1
Practice - 3.4 1, 1, 1
Practice - 3.4
Practice - 3.4
Practice - 3.4
1. Find the HCF of the following by factorisation method.

a. 225 and 450 b. 135 and 165 c. 144 and 312

d. 531, 726 and 984 e. 405, 426 and 507 f. 255, 340 and 765

2. Find the HCF of the following numbers by finding their common factors.

a. 28 and 48 b. 24 and 50 c. 30 and 75

d. 8, 12 and 40 e. 36 and 60 f. 48 and 72

3. Find the HCF of the following numbers.

a. 1035 and 1173 b. 465 and 555 c. 1131 and 899

Mathematics-5 60