Page 62 - Maths Class 05

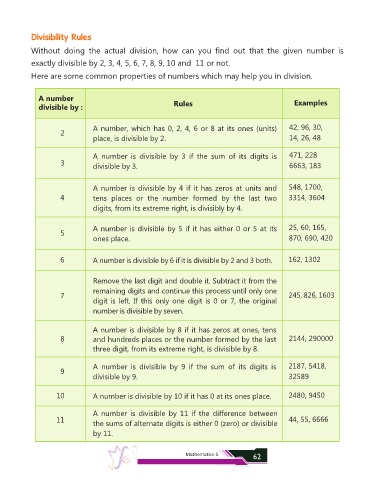
Divisibility Rules

Without doing the actual division, how can you find out that the given number is

exactly divisible by 2, 3, 4, 5, 6, 7, 8, 9, 10 and 11 or not.

Here are some common properties of numbers which may help you in division.

A number
Rules Examples
divisible by :

A number, which has 0, 2, 4, 6 or 8 at its ones (units) 42, 96, 30,
2
place, is divisible by 2. 14, 26, 48

A number is divisible by 3 if the sum of its digits is 471, 228
3
divisible by 3. 6663, 183

A number is divisible by 4 if it has zeros at units and 548, 1700,
4 tens places or the number formed by the last two 3314, 3604
digits, from its extreme right, is divisibly by 4.

A number is divisible by 5 if it has either 0 or 5 at its 25, 60, 165,
5
ones place. 870, 690, 420

6 A number is divisible by 6 if it is divisible by 2 and 3 both. 162, 1302

Remove the last digit and double it. Subtract it from the
remaining digits and continue this process until only one
7 245, 826, 1603
digit is left. If this only one digit is 0 or 7, the original
number is divisible by seven.

A number is divisible by 8 if it has zeros at ones, tens
8 and hundreds places or the number formed by the last 2144, 290000

three digit, from its extreme right, is divisible by 8.

A number is divisible by 9 if the sum of its digits is 2187, 5418,
9
divisible by 9. 32589

10 A number is divisible by 10 if it has 0 at its ones place. 2480, 9450

A number is divisible by 11 if the difference between
11 44, 55, 6666
the sums of alternate digits is either 0 (zero) or divisible
by 11.

Mathematics-5 62